

Department of History
University of California, Irvine
Instructor: Dr. Barbara J. Becker
![]()
Lecture 6. Uncovering the Cosmic Blueprint.
![]()
| Johannes Kepler (1571-1630) |
|
He was constantly on the move, ferreting among the sciences, politics and private affairs, including the lowest kind; always following someone else, and imitating his thoughts and actions.... [H]e grieved that on account of the impurity of his life, the honor to be a prophet was denied him.... In this man there are two opposite tendencies: always to regret any wasted time, and always to waste it willingly.... |
 |
|
|
Notable Events in Kepler's Life |
|
1571 (May 16, 4.37 am) |
conceived |
1571 (Dec 27, 2.30 pm) |
born at Weil-der-Stadt |
1589 |
entered University of Tubingen |
1591 |
received Master's degree began work as mathematics instructor at Gratz |
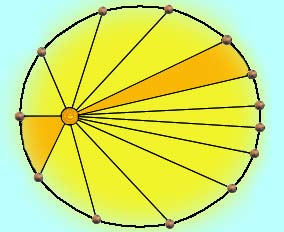
| excerpt from There were three things in particular about which I persistently sought the reasons why they were such and not otherwise: the number, the size, and the motion of the circles.... In the beginning I attacked the business by numbers, and considered whether one circle was twice another, or three times, or four times, or whatever, and how far any one was separated from another according to Copernicus. I wasted a great deal of time on that toil, as if at a game, since no agreement appeared either in the proportions themselves or in the differences; and I derived nothing of value from that except that I engraved deeply on my memory the distances which were published by Copernicus.... If (thought I) God allotted motions to the spheres to correspond with their distances, similarly he made the distances themselves correspond with something....
|
At roughly twenty year intervals, Jupiter and Saturn appear very close to one another on the sky. Astronomers call this a "conjunction". One day, while teaching a lesson on planetary conjunctions to his students at Gratz, Kepler drew a diagram to illustrate a series of conjunctions of Jupiter and Saturn. A pattern emerged that he believed was a key to the "cosmic mystery". Here's an example of the data Kepler used to make his diagram. The table (right) lists the constellations Jupiter and Saturn appeared in at the time of their conjunctions from 1300-1490. |
Conjunctions of Saturn and Jupiter (1300-1490)
|
||||||||||||||||||||||||||||||||||
Conjunctions of Saturn and Jupiter (1300-1490)
|
|||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||
Conjunctions of Saturn and Jupiter (1570-1770) |
|||||||||||||||||||||||||||||||||||
|
|||||||||||||||||||||||||||||||||||
Conjunctions of Saturn and Jupiter (1570-1770)
|
|||||||||||||||||||||||||||||||||||
Continuing to plot Jupiter-Saturn conjunctions over 40 cycles generates the pattern seen below. Kepler was struck by the emergence of what appeared to be a smaller circle inscribed within the larger original one. Could this be the mathematical basis of the natural spacing between the planets?
|
|||||||||||||||||||||||||||||||||||
Kepler's Mysterium Cosmigraphicum (1596) continued....
Eventually by a certain mere accident I chanced to come closer to the actual state of affairs. I thought it was by divine intervention that I gained fortuitously what I was never able to obtain by any amount of toil; and I believed that all the more because I had always prayed to God that if Copernicus had told the truth things should proceed in this way.... I naturally concluded that by this method if I wished to keep an order among the figures, I should never reach the Sun, nor have an explanation why there should be six moving circles rather than twenty or a hundred. However, the figures were satisfactory, as they represented quantities, and so something prior to the heavens. For quantity was created in the beginning along with matter, but the heavens on the second day. But if (thought I) corresponding with the size and proportion of the six heavens, as Copernicus established them, there could be found only five figures, among the infinite number of others, which had certain special properties distinct from the rest, it would be the answer to my prayer. Again I set to. Why should there be plane figures between solid spheres? It would be more appropriate to try solid bodies. Behold, reader, this is my discovery and the subject matter of the whole of this little work. For if anyone having a slight acquaintance with geometry were informed of this in so many words, there would immediately come to his mind the five regular solids with the proportion of their circumscribed spheres to those inscribed.... This accident was also the happy ending of my toil. You can now also see my scheme for this book. What delight I have found in this discovery I shall never be able to express in words. No longer did I regret the wasted time; I was no longer sick of the toil; I did not avoid any of the tedium of the calculation; I devoted my days and nights to computation, until such time as I could see whether the proposition which I had conceived in words would agree with the circles of Copernicus, or whether my joy would be scattered to the winds. But if I found out that I was right, I made a vow to Almighty God that at the first opportunity I would proclaim among men in public print this wonderful example of his wisdom....
|
Notable Events in Kepler's Life (cont'd) |
|
| 1595 | formulated "fourth law"--
six planetary orbits are nested in five Platonic solids |
|
|
||||||||||||||||||||||
Notable Events in Kepler's Life (cont'd) |
|||
| 1599 | accepted a position at Prague with Tycho | ||
| 1600 | began work on problem of Mars's orbit | ||
| 1601 | death of Tycho; Kepler acquired Tycho's observation records | ||
| 1601-3 | used Tycho's observations to launch what he called his "battle with Mars" with the goal of establishing with certainty the central position of the Sun in the celestial system soon abandoned the notion of celestial bodies having to move at constant speeds: planets move faster when they are closer to the Sun, slower when they are far away
formulated "second law"--
|
||
|
|||
| 1603 | abandoned the notion of celestial bodies having to move in perfect circles Kepler first assumed that planets follow an oval path. He modified the oval to an egg-shape. Only after a very long struggle with the problem did he conclude that the shape of planetary orbits is that of an ellipse: formulated "first law"--
|
||
Kepler on Elliptical Orbits Why should I mince my words? The truth of Nature, which I had rejected and chased away, returned by stealth through the backdoor, disguising itself to be accepted. That is to say, I laid [the original equation] aside, and fell back on ellipses, believing that this was quite a different hypothesis, whereas the two ... are one and the same. I thought and searched, until I went nearly mad, for a reason why the planet preferred an elliptical orbit [to mine].... Ah, what a foolish bird I have been! |
|||
Notable Events in Kepler's Life (cont'd) |
|||
| 1604 | observed a New Star (known today as "Kepler's Star") in the constellation Ophiuchus
Star map showing Kepler's Star (highlighted in yellow) |
||
| 1606 | published De Stella Nova (On the New Star)
The illustration's caption reads |
||
| 1609 | published Astronomia Nova (New Astronomy)
|
||
Kepler on Force that Controls Earth's Orbital Motion It is therefore clear that the traditional doctrine about gravity is erroneous.... Gravity is the mutual bodily tendency between like bodies towards unity or contact (of which kind the magnetic force also is), so that the earth draws a stone much more than the stone draws the earth.... Suppose the earth were in the center of the world. Heavy bodies would be attracted to it, not because it is in the center, but because it is a like body. It follows that regardless where we place the earth ... heavy bodies will always seek it.... |
|||
Notable Events in Kepler's Life (cont'd) |
|
| 1618 | formulated "third law"--
T2/R3 = constant, for all satellites orbiting any given central body |
| 1619 | published Harmonice Mundi (Harmony
of the World)
|
| 1621 | published Epitome Astronomiæ Copernicanæ (Epitome
of Copernican Astronomy)
|
| 1627 | published Rudolphine Tables, the first astronomical tables based on Kepler's new system of elliptical orbits |
Frontispiece for the Rudolphine Tables (1627) designed by Kepler himself. The frontispiece shows a temple dedicated to Urania (she is seated on top of the dome holding a laurel wreath). An imperial eagle flies above the dome. Gold coins fall from the eagle's beak down to the hard-working astronomers below. Six figures stand along the edge of the dome representing the useful tools available to the modern astronomer: (left to right) the laws of optics, the telescope, logarithms, geometry, physical laws of balance, and the lodestone and compass. The base is decorated with panels depicting the origins of the Rudolphine Tables. On the far left is a portrait of Rudolph II -- the Holy Roman Emperor who commissioned Tycho to create the Tables -- next is portrait of Kepler designing the temple, in the middle is a map of the island of Hven in Denmark where Tycho built his famous observatory castle Uraniborg, to the right we see the publisher assembling the book, and the figure in the panel on the far right appears to be studying the Tables. The floor of the Temple is a map of the heavens, the foundation of astronomy on which all the astronomers stand. The dome is supported by ten pillars. At the back are two bare tree trunks. They are very crude and do not quite reach the ceiling -- pieces of wood are wedged in at the top to keep them in place. They represent the ancient history of astronomy. A Babylonian astronomer stands near them. He has no sighting instruments so relies on his hands to estimate the positions of celestial bodies. Stacks of roughly-hewn stone form the next two pillars. Four brick pillars with many cracks and patches have early astronomical instruments (armillary sphere, celestial sphere, astrolabe, and lunar tables) hanging on them. Hipparchus stands next to the front brick pillar on the left. Ptolemy sits next to the front pillar on the right. Two finely-crafted marble pillars memorialize the work of Copernicus and Tycho Brahe. Pointing upward with his right hand, Tycho draws the attention of Copernicus to the ceiling which is decorated with an illustration of Tycho's geo-heliocentric system. His left hand points down toward the starry map on the floor. He asks "Quid si sic?" -- "Is it thus?" Tycho seems genuinely puzzled to see the title of the book hanging down from a cord emerging from the Sun's position on the diagram, not the Earth. Perhaps Kepler has designed the illustration in this way to show that the Tables are based on a Sun-centered system. |
|
|
Kepler viewed himself as a "Copernican." What did he mean by that? Would you classify Kepler's work as "scientific"? What was the Copernican Revolution? In 1600, what did it mean to say that one was a Copernican? A moving earth required a new physics. A new physics required--
|
|